Question

In triangle ABC, AC =12, the measure of angle A = 30, and the measure of angle B = 45. Find the area of this triangle. (Hint: Draw a picture and include the altitude from vertex C to the side AB.)

(A) 18√3
(B) 54√ 3
(C) 18(1 + √ 3)
(D) 54(1 + √ 3)
(E) 12(1 + √ 3)
(F) none of the above

Answer 1

(C) 18(1 + √ 3)

Explanation:

Given,

In triangle ABC,

AC = 12 unit,

m∠A = 30°,

m∠B = 45°,

Let D∈AB such that CD ⊥ AB,

In triangle ADC,

`sin A = (CD)/(AC)`

`sin 30^(\circ)=(CD)/(12)`

`(1)/(2)=(CD)/(12)`

`\implies CD=6\text{ unit}`,

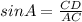
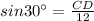
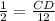
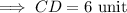By the pythagorean theorem,

`AC^2=CD^2+AD^2\implies 144 = 36 + AD^2\implies 108=AD^2\implies AD=6√(3)\text{ unit}`

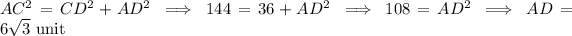
Now, in triangle CDB,

`tan 45^(\circ)=(CD)/(DB)`

`1=(6)/(DB)\implies DB=6\text{ unit}`

`AB=AD+DB=6√(3)+6=6(√(3)+1)\text{ unit}`

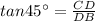
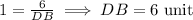
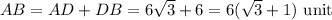
Hence, the area of the triangle ABC =
`(1)/(2)* AB* CD`

`=(1)/(2)* 6(√(3)+1)* 6`

`=18(√(3)+1)\text{ square unit}`

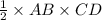
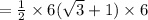
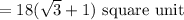
Option 'C' is correct.