Question

Silver chloride, AgCl (Ksp = 1.8 x 10‒10), can be dissolved in solutions containing ammonia due to the formation of the soluble complex ion Ag(NH3)2 + (Kf = 1.0 x 108 ). What is the minimum amount of NH3 that would need to be added to dissolve 0.010 mol AgCl in 1.00 L of solution?

Answer 1

Step-by-step explanation:

The given reaction will be as follows.

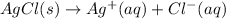`AgCl(s) \rightarrow Ag^(+)(aq) + Cl^(-)(aq)` ............. (1)

`K_(sp)` =
`[Ag^(+)][Cl^(-)] = 1.8 * 10^(-10)`

Reaction for the complex formation is as follows.

`Ag^(+)(aq) + 2NH_(3)(aq) \rightleftharpoons [Ag(NH_(3))_(2)]^(+)(aq)` ........... (2)

`K_(f)` =
`([Ag(NH_(3))_(2)])/([Ag^(+)][NH_(3)]^(2)) = 1.0 * 10^(8)`

When we add both equations (1) and (2) then the resultant equation is as follows.

`AgCl(s) + 2NH_(3)(aq) \rightarrow [Ag(NH_(3))_(2)]^(+)(aq) + Cl^(-)(aq)` ............. (3)

Therefore, equilibrium constant will be as follows.

K =
`K_(f) * K_(sp)`

=
`1.0 * 10^(8) * 1.8 * 10^(-10)`

=
`1.8 * 10^(-2)`

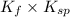
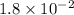
Since, we need 0.010 mol of AgCl to be soluble in 1 liter of solution after after addition of
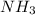`NH_(3)` for complexation. This means we have to set

`[Ag^(+)]` =
`[Cl^(-)]`

=
`(0.010 mol)/(1 L)`

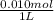
= 0.010 M

For the net reaction,
`AgCl(s) + 2NH_(3)(aq) \rightarrow [Ag(NH_(3))_(2)]^(+)(aq) + Cl^(-)(aq)`

Initial : 0.010 x 0 0

Change : -0.010 -0.020 +0.010 +0.010

Equilibrium : 0 x - 0.020 0.010 0.010

Hence, the equilibrium constant expression for this is as follows.

K =
`([Ag(NH_(3))^(+)_(2)][Cl^(-)])/([NH_(3)]^(2))`

`1.8 * 10^(-2)` =
`(0.010 * 0.010)/((x - 0.020)^(2))`

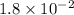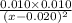
x = 0.0945 mol

or, x = 0.095 mol (approx)

Thus, we can conclude that the number of moles of
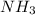`NH_(3)` needed to be added is 0.095 mol.