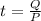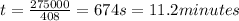Question

Older freezers developed a coating of ice inside that had to be melted periodically; an electric heater could speed this defrosting process. Suppose you're melting ice from your freezer using a heating wire that carries a current of 3.4 when connected to 120 .What is the resistance of the wire?R=How long will it take the heater to melt 780 of accumulated ice at -10 ? Assume that all of the heat goes into warming and melting the ice, and that the melt water runs out and doesn't warm further.t=

Answer 1

R = 35.3 ohms

It would take 11.2 minutes

Step-by-step explanation:

Ohm's law states that

`R = (V)/(I)`

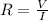
Therefore:

`R = (120 V)/(3.4 A) = 35.3 ohms`

Joule's law states that

`P = I^2 * R`

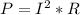
So

`P = 3.4^2 * 35.3 = 408 w`

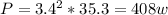
I assume the problem means 780 g of ice.

The specific heat capacity of ice is:

Cp = 2 kJ/(kg*K)

And the latent heat to melt ice is:

Cl = 333 kJ/kg

So, the heat needed to melt ice from -10 C is:

Q = m * Cp * (tfinal - ti) + m * Cl

Q = 0.78 * (2 * (0 - (-10)) + 333) = 275 kJ

Power is energy over time

`P = (Q)/(t)`

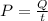
so with a power of P it would take t seconds to deliver an energy Q

`t = (Q)/(P)`

`t = (275000)/(408) = 674 s = 11.2 minutes`