Answer:
The area increase by 225%
Step-by-step explanation:
attachment of a triangle
The area of a triangle equilateral is calculated with the next formula:
A=
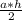
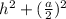 =
=
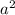
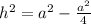 =
=
 *3
*3
h=
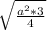
h=
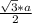
replacing in the A equation:
A=
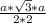
A=
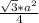
Now each side increse by 50% ⇒ a=1.5a
A=
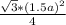
A=
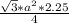
A=2.25 *
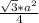
It means that the area incrase by 225%