Answer:
The largest angle of the quadrilateral is 120°.
Explanation:
Given : The ratio of three consecutive angles in a cyclic quadrilateral is 2:3:4
To find : The largest angle of the quadrilateral, in degrees ?
Solution :
Let the ration be 'x',
So, The consecutive angles are 2x , 3x and 4x.
We know that, Opposite angles of cyclic quadrilateral is 180°.
i.e.
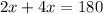
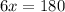
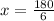
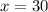
The angles became,
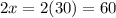
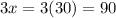
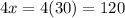
We know that sum of all angles of quadrilateral is 360°,
Let the fourth angle be A,
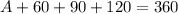

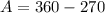
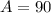
Therefore, Among all the angles the largest angle of the quadrilateral is 120°.