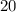Question

Customers of a phone company can choose between two service plans for long distance calls. The first plan has no monthly fee but charges for each minute of calls. The second plan has a monthly fee and charges an additional for each minute of calls. For how many minutes of calls will the costs of the two plans be equal?

Answer 1

\\x= P/(c -d)[/tex],

Assume that the price of each minute in the first plan is $c and that the second plan charges a flat rate of $P and a charge of additional $d for every minute.

Explanation

Assume that the price of each minute in the first plan is $c and that the second plan charges a flat rate of $P and a charge of additional $d for every minute.

Thus, the monthly cost of a customer who consumes x minutes in each plan is:

For the first plan:
`cx`

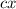
and for the second plan:
`P + dx`

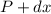
Considering that the monthly costs must be the same in each plan, you have to:

`cx = P + dx\\ transposing terms</p><p>\\cx - dx = P\\   applying common factor</p><p>\\(c -d)x = P\\ dividing by [tex]c - d`

\\x= P/(c -d)[/tex].

For example if
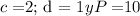`c = $2; d = $1 y P = $10`, Then the number of minutes would be,
`x=10` and the total cost for each plan would be
`$20`