Question

What is the domain of the function?
f(x) square root of x+1 over (x+4)(x-6)

Answer 1

[-1,6)U(6, ∞)

Explanation:

`f(x) = (√(x+1))/((x+4)(x-6))\\`

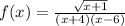
In functions that have a square root, the radicand (the inside of the root) has to be ≥0. Otherwise, you would be dealing with imaginary numbers.

So, in our function,

`x+1 \geq0\\x\geq-1`

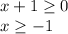
We have two factors in our denominator. Note that you cannot divide by 0. So to find where x cannot be, we have to set the denominator to 0.

`(x+4)(x-6)=0\\x+4=0 \\x-6 = 0\\\\x=-4\\x=6`

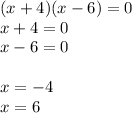
X cannot be either -4 or 6.

So, x has to be greater than or equal to -1 and cannot equal -4 or 6.

x≥-1, x≠6