Question

How many grams of Sg is required to produce 83.10 g SF6? S: +24F-->8SF

Answer 1

Answer : The mass of
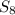`S_8` required is 18.238 grams.

Explanation : Given,

Mass of
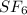`SF_6` = 83.10 g

Molar mass of
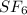`SF_6` = 146 g/mole

Molar mass of
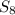`S_8` = 256.52 g/mole

The balanced chemical reaction is,

`S_8+24F_2\rightarrow 8SF_6`

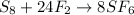
First we have to determine the moles of
`SF_6`.

`\text{Moles of }SF_6=\frac{\text{Mass of }SF_6}{\text{Molar mass of }SF_6}=(83.10g)/(146g/mole)=0.569moles`

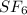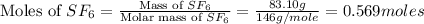
Now we have to determine the moles of
`S_8`.

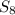From the balanced chemical reaction we conclude that,

As, 8 moles of
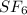`SF_6` produced from 1 mole of
`S_8`

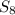
So, 0.569 moles of
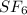`SF_6` produced from
`(0.569)/(8)=0.0711` mole of
`S_8`

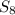
Now we have to determine the mass of
`S_8`.

`\text{Mass of }S_8=\text{Moles of }S_8* \text{Molar mass of }S_8`

`\text{Mass of }S_8=(0.0711mole)* (256.52g/mole)=18.238g`

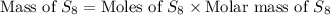
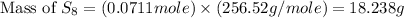
Therefore, the mass of
`S_8` required is 18.238 grams.