Answer:
Explanation:
Given is a differential equation
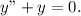
SInce it is a power series solution let us assume
![y =[tex]}](https://img.qammunity.org/2020/formulas/mathematics/college/l41ieikhpsv9ra5am2rnd78nxp2ce17l4c.png) [/tex]
[/tex]
Find I and II derivative


Now substitute in the given DE
2a_2 +3(2)a_3x^2+...+a_n n(n-1)x^{n-2} +... a_0+a_1x+....+a_nx^n +....=0\\\\a_{n-2} +n(n-1) a_n =0\\a_n = \frac{-a_{n-2}}{n(n-1)
Thus the solution is a power series with recurring formula
a_n = \frac{-a_{n-2}}{n(n-1)