Answer:
Step-by-step explanation:
The relation between the de Broglie wavelength and the momentum of the particle is given by
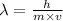
where, m is the mas of the particle and v be the velocity of the particle and h be the Plank's constant.
So, the de broglie wavelength of proton is given by
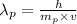 .... (1)
.... (1)
The de broglie wavelength of electron is given by
 .... (2)
.... (2)
Divide equation (2) by equation (1), we get

As the mass of proton is much more than the mass of electron, so the de broglie wavelength of electron is more than the de Broglie wavelength of proton.