Answer:
The distance and height of the object is 6 m and 2 m.
The image is virtual and upright.
Step-by-step explanation:
Given that,
Focal length = 0.25 m
Length of image = 0.080 m
Image distance = 0.24 m
We need to calculate the distance of the object
Using formula of lens
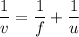
Put the value into the formula
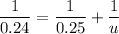
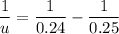
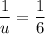
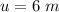
We need to calculate the magnification
Using formula of magnification
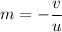
Put the value into the formula
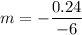
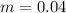
We need to calculate the height of the object
Using formula of magnification
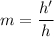
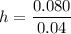
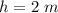
A convex mirror produce a virtual and upright image behind the mirror.
Hence, The distance and height of the object is 6 m and 2 m.
The image is virtual and upright.