Answer:
It will take 20 years for the size of the population to reach 150% of its current size according to the exponential growth function.
Step-by-step explanation:
The exponential growth function is given by:
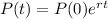
In which
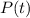 is the population after t years,
is the population after t years,
 is the initial population, e is the Euler number and r is the growth rate(decimal).
is the initial population, e is the Euler number and r is the growth rate(decimal).
In this problem, we have that:
A population of kangaroos is growing at a rate of 2% per year, compounded continuously. This means that
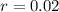 .
.
If the growth rate continues, how many years will it take for the size of the population to reach 150% of its current size according to the exponential growth function?
This is the value of t when
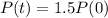 . So
. So
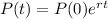

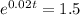
Since ln and e are inverse operation, we apply e to both sides of the equation to find t.

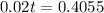
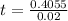
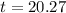
Rouding to the nearest whole number, it is 20 years.
It will take 20 years for the size of the population to reach 150% of its current size according to the exponential growth function.