Answer:
3.5
Explanation:
We can use the cos rule to solve this type of problem. The cos rule is given by:

Where p is the side opposite of the "in-between" angle given and P is the angle given between 2 sides. Also, a and b are the 2 sides given (adjacent to the angle).
So, here, "p" is what we want to find (smallest side is opposite of smallest angle). And, a and b are 4 & 7 respectively. P is 20 degrees.
Now, using Cos Rule:
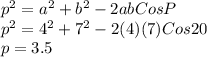
So, the smallest side, p, is 3.5