Question

Water at a pressure of 3 bars enters a short horizontal convergent channel at 3.5 m/s. The upstream and downstream diameters of the channel are 50 mm and 30 mm respectively. Determine the downstream pressure if energy losses are neglected.

Answer 1

The pressure reduces to 2.588 bars.

Step-by-step explanation:

According to Bernoulli's theorem for ideal flow we have

`(P)/(\gamma _(w))+(V^(2))/(2g)+z=constant`

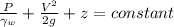
Since the losses are neglected thus applying this theorm between upper and lower porion we have

`(P_(u))/(\gamma _(w))+\frac{V-{u}^(2)}{2g}+z_(u)=(P_(L))/(\gamma _(w))+\frac{V{L}^(2)}{2g}+z_(L)`

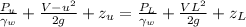
Now by continuity equation we have

`A_(u)v_(u)=A_(L)v_(L)\\\\\therefore v_(L)=(A_(u))/(A_(L))* v_(u)\\\\v_(L)=(d^(2)_(u))/(d^(2)_(L))* v_(u)\\\\\therefore v_(L)=(2500)/(900)* 3.5\\\\\therefore v_(L)=9.72m/s`

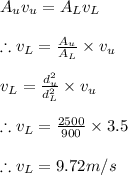
Applying the values in the Bernoulli's equation we get

`(P_(L))/(\gamma _(w))=(300000)/(\gamma _(w))+(3.5^(2))/(2g)-(9.72^(2))/(2g)(\because z_(L)=z_(u))\\\\(P_(L))/(\gamma _(w))=26.38m\\\\\therefore P_(L)=258885.8Pa\\\\\therefore P_(L)=2.588bars`