Answer:
A ≈ 8133.27 m²
Explanation:
Sum the parts of the ratio 6 + 7 + 8 = 21 parts
Divide the perimeter by 21 to find the value of one part
420 ÷ 21 = 20 ← value of 1 part of the ratio, hence sides of triangle are
6 × 20 = 120
7 × 20 = 140
8 × 20 = 160
To calculate the area (A) we can use Hero's formula
A =
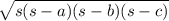
Where s is the semi perimeter and a, b, c the sides of the triangle
let a = 120, b = 140 , c = 160 and s = 420 ÷ 2 = 210
A =
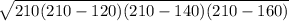
=
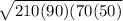
=
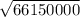 ≈ 8133.27 m²
≈ 8133.27 m²