Question

The density of liquid mercury is 13.5 g/cm^3. What mass of mercury (in kg) is required to fill a hollow cylinder having an inner diameter of 2.00 cm to a height of 25.0 cm? *

Answer 1

Answer: 1.06 kg

Step-by-step explanation:

Density is defined as the mass contained per unit volume.

`Density=(mass)/(Volume)`

Given :

Density of mercury =
`13.5g/cm^3`

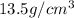
volume of cylinder = volume of mercury =
`\pir^2h`

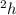
where r = radius of cylinder =
`(diameter)/(2)=(2cm)/(2)=1cm`

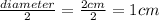
h= height = 25.0 cm

Putting in the values we get:

volume of mercury =
`3.14* 1^2* 25=78.5cm^3`

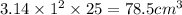
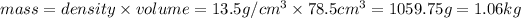`mass=density* volume=13.5g/cm^3* 78.5cm^3=1059.75g=1.06kg` (1kg=1000g)

Thus mass of mercury required to fill a hollow cylinder having an inner diameter of 2.00 cm to a height of 25.0 cm is 1.06 kg.