Answer:
The value of k = 4/3
Explanation:
* Lets explain how to solve the problem
- An equilateral triangle ABC is inscribed in a circle N
- The area of the triangle is √3
- The shaded area is the difference between the area of the circle
and the area of the equilateral triangle ABC
- The shaded are = k π - √3
- We need to find the value of k
* At first lets find the length of the side of the Δ ABC
∵ Δ ABC is an equilateral triangle
∴ Its area = √3/4 s² , where s is the length of its sides
∵ The area of the triangle = √3
∴ √3/4 s² = √3
- divide both sides by √3
∴ 1/4 s² = 1
- Multiply both sides by 4
∴ s² = 4 ⇒ take √ for both sides
∴ s = 2
∴ The length of the side of the equilateral triangle is 2
* Now lets find the radius of the circle
- In the triangle whose vertices are A , B and N the center of the circle
∵ AN and BN are radii
∴ AN = BN = r , where r is the radius of the circle
∵ The sides of the equilateral angles divides the circle into 3 equal
arcs in measure where each arc has measure 360°/3 = 120°
∵ The measure of the central angle in a circle equal the measure
of the its subtended arc arc
∵ ∠ANB is an central angle subtended by arc AB
∵ The measure of arc AB is 120°
∴ m∠ANB = 120°
- By using the cosine rule in Δ ANB
∵ AB = 2 , AN = BN = r , m∠ANB = 120°
∴
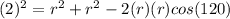
∴
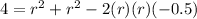
∴
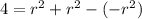
∴
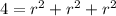
∴
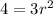
- Divide both sides by 3
∴
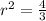
- Take square root for both sides
∴ r = 2/√3
* Lets find the value of k
∵ Area circle = πr²
∵ r = 2/√3
∴ Area circle = π(2/√3)² = (4/3)π
∵ Area shaded = area circle - area triangle
∵ Area triangle = √3
∴ Area shaded = (4/3) π - √3
∵ Area of the shaded part is π k - √3
- Equate the two expressions
∴ π k - √3 = (4/3) π - √3
∴ k = 4/3
* The value of k = 4/3