Answer:
The graph of f(x) stretch vertically by factor 2, shifts 7 units right and 95 units down to get the graph of g(x).
Explanation:
The given functions are
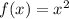
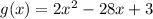
Rewrite the function g(x) in vertex form.
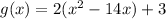
If an expression is defined as
 then we need to add
then we need to add
 to make it perfect square.
to make it perfect square.
In the above parenthesis b=-14. So add 7² in the parenthesis.
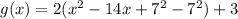
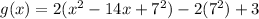
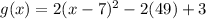
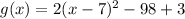
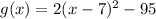 .... (1)
.... (1)
The translation is defined as
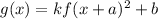 .... (2)
.... (2)
Where, k is stretch factor, a is horizontal shift and b is vertical shift.
If 0<k<1, then the graph compressed vertically by factor k and if k>1, then the graph stretch vertically by factor k.
If a>0, then the graph shifts a units left and if a<0, then the graph shifts a units right.
If b>0, then the graph shifts b units up and if b<0, then the graph shifts b units down.
On comparing (1) and (2), we get

It means the graph of f(x) stretch vertically by factor 2, shifts 7 units right and 95 units down to get the graph of g(x).