Question

The distribution of the amount of a certain brand of soda in 16 OZ bottles is approximately normal with a mean of 16.12 OZ and a standard deviation of 0.09 OZ. The percentage of the soda bottles that contain more than the 16 OZ advertised is: _______%

Answer 1

Answer: 90.82%

Explanation:

Given : The distribution of the amount of a certain brand of soda in 16 OZ bottles is approximately normal .

Mean :
`\mu=16.12\text{ OZ}`

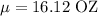
Standard deviation:
`\sigma=0.09\text{ OZ}`

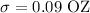
Let X be the random variable that represents the amount of soda in bottles.

Formula for z-score :
`z=(x-\mu)/(\sigma)`

Z-score for 16 oz:
`z=(16-16.12)/(0.09)=-1.33`

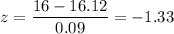
Using the standard normal z-distribution table , the probability that the soda bottles that contain more than the 16 OZ is given by :_

`P(x>60)=P(z>-1.33)=1-P(x\leq-1.33)=1-0.0917591=0.9082409\approx0.9082\approx90.82\%`

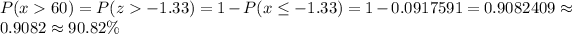
Hence, the percentage of the soda bottles that contain more than the 16 OZ advertised is 90.82% .