Answer:
The total angular momentum of these hands is 1.20 kg m²/s.
Step-by-step explanation:
Given that,
Length of hour hand = 2.70 m
Length of minute hand = 4.50 m
Mass of hour hand = 60.0 kg
Mass of minute hand = 100 kg
We need to calculate the total angular momentum
Using formula of angular momentum
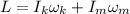
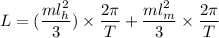
Where,
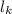 = length of hour hand
= length of hour hand
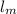 =length of minute hand
=length of minute hand
Put the value into the formula

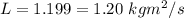
Hence, The total angular momentum of these hands is 1.20 kg m²/s.