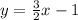Question

Write the equation of a line in slope-intercept form passing through (4, 5) and (6, 8)

Answer 1

Answer:
`y=(3)/(2)x-1`

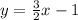
Explanation:

The equation of the line in Slope-Intercept form is:

`y=mx+b`

Where "m" is the slope and "b" is the y-intercept.

Knowing that this line passes through the points (4, 5) and (6, 8), we can find the slope with the formula
`m=(y_2-y_1)/(x_2-x_1)`. Then:

`m=(5-8)/(4-6)=(3)/(2)`

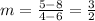
Substitute one point and the slope into
`y=mx+b` and solve for "b":

`5=(3)/(2)(4)+b\\\\5-6=b\\\\b=-1`

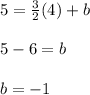
Then, the equation of this line in Slope-Intercept form is:

`y=(3)/(2)x-1`

Answer 2

y = 3/2 x-1 ..

Explanation:

First find the slope.

m = y2-y1/x2-x1

x1 = 4 , x2 = 6

y1 = 5, y2 = 8

m = 8-5/ 6-4

m = 3/2

Slope intercept form of linear equation:

y = mx+b

5 = 3/2 * 4 +b

5 = 12/2 +b

5 = 12+2b/2

10 = 12+2b

10-12 = 2b

-2 = 2b

Divide both sides by 2.

-2/2 = 2b/2

-1 = b

Thus slope intercept form of equation is:

y = 3/2 x-1 ....