Question

A stone is dropped into a well. The sound of the splash is heard 3.5 seconds later. What is the depth of the well? Take the speed of sound in air to be 343 m/s.

Answer 1

The depth of the well, s = 54.66 m

Given:

time, t = 3.5 s

speed of sound in air, v = 343 m/s

Solution:

By using second equation of motion for the distance traveled by the stone when dropped into a well:

`s = ut +(1)/(2)at^(2)`

Since, the stone is dropped, its initial velocity, u = 0 m/s

and acceleration is due to gravity only, the above eqn can be written as:

`s = (1)/(2)gt'^(2)`

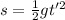
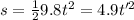`s = (1)/(2)9.8t^(2) = 4.9t'^(2)` (1)

Now, when the sound inside the well travels back, the distance covered,s is given by:

`s = v* t''`

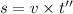
`s = 343* t''` (2)

Now, total time taken by the sound to travel:

t = t' + t''

t'' = 3.5 - t' (3)

Using eqn (2) and (3):

s = 343(3.5 - t') (4)

from eqn (1) and (4):

`4.9t'^(2) = 343(3.5 - t')`

`4.9t'^(2) + 343t' - 1200.5 = 0`

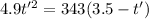
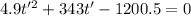
Solving the above quadratic eqn:

t' = 3.34 s

Now, substituting t' = 3.34 s in eqn (2)

s = 54.66 m