Question

Which statement is true about the graphs of the two lines y= -8x - 5/4 and y = 1/8 x + 4/5?

Answer 1

The lines are perpendicular.

Explanation:

`\text{The slope-intercept form of an equation of a line:}\\\\y=mx+b\\\\m-slope\\b-y-intercept\\\\\text{Let}\ k:y=m_1x+b_1,\ l:y=m_2x+b_2\\\\k\ \parallel\ l\iff m_1=m_2\\\\k\ \perp\ l\iff m_1m_2=-1\to m_2=-(1)/(m_1)`

`\text{We have}\\\\y=-8x-(5)/(4)\to m_1=-8\\\\y=(1)/(8)x+(4)/(5)\to m_2=(1)/(8)\\\\m_1\\eq m_2-\text{given lines are not parallel}\\\\m_1m_2=-8\left((1)/(8)\right)=-1-\text{given lines are perpendicular}`

Answer 2

`y= -8x - (5)/(4)`

`y= (1)/(8)x + (4)/(5)`

↓

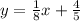
The lines are perpendicular

Explanation:

If we have two lines of equations:

`y = nx + v` (1)

`y = mx + b` (2)

Where n is the slope of the line (1) and m is the slope of the line (2)

Then by definition it is fulfilled that if
`n = -(1)/(m)` means that the lines (1) and (2) are perpendicular

In this case the lines are:

`y= -8x - (5)/(4)` (1)

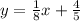`y= (1)/(8)x + (4)/(5)` (2)

Observe that:
`n=-8` and
`m=(1)/(8)`

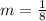
Then it is fulfilled that
`n = -(1)/(m)` because:

`n =-(1)/((1)/(8))`

`n = -8`

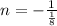
Therefore the lines are perpendicular