Answer:
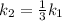
Solution:
Natural frequency of a spring mass system is given by:
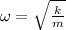 (1)
(1)
Now, with a system with
 and mass m, natural frequency is:
and mass m, natural frequency is:
 (2) (given)
(2) (given)
Also, when another spring
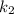 is added in series with the first one the natural frequency of the system reduces to
is added in series with the first one the natural frequency of the system reduces to
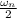 , spring's equivalent stiffness is given by:
, spring's equivalent stiffness is given by:
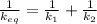
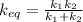
Therefore,
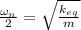
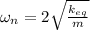 (3)
(3)
From eqn (2) and (3):
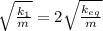
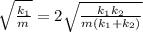
Squaring both sides of the above eqn, we get:
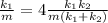
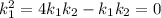
Solving the above equation in order to get the relation between
 and
and
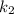 :
:
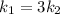
Therefore,
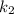 in terms of
in terms of
 :
: