Question

A 54 gram coin sits on a horizontally rotating turntable. The turntable makes 1.0 revolution each second. The coin is located 10 cm from the axis of rotation of the turntable. The coin will slide off the turntable if it is located more than 14.4 cm from the axis of rotation. Calculate the coefficient of static friction? please show all the work

Answer 1

`\mu = 0.578`

Step-by-step explanation:

given data:

revolution per second
`\omega  = 1 rev/s = 1 * 2\pi = 6.28 rad/s`

for sliding off

maximum static friction
`= m \omega^2 * r`

maximum static friction is frictional force, hence we have

`\mu mg = m \omega^2 r`

solving for \mu

`\mu = (\omega^2 r)/(g)`

`\mu = (6.28^2 * .144)/(9.81)`

`\mu = 0.578`

Answer 2

The coefficient of static friction is 0.578.

Step-by-step explanation:

Given that,

Mass of coin = 54 gm

Revolution r = 1.0

Distance = 14.4 cm

We need to calculate the force when the coin located more than 14.4 cm from the axis of rotation

Using formula of centripetal force

`F= mR\omega^2`

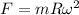
Where, m = mass

R = radius

Put the value into the formula

`F=0.054*10*14.4^(-2)*(2\pi)^2`

`F=0.306\ N`

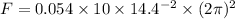
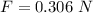
We need to calculate the coefficient of static friction

Using formula of friction

`F=\mu mg`

`\mu=(F)/(mg)`

`\mu=(0.306)/(0.054*9.8)`

`\mu=0.578`

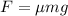
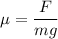
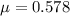
Hence, The coefficient of static friction is 0.578.