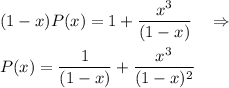Question

Find the generating function for the sequence 1,1,1,2,3,4,5,6,....

Answer 1

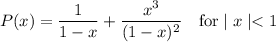`P(x)=(1)/(1-x)+(x^3)/((1-x)^2) \quad \text{for} \mid x \mid < 1`[/tex]

Explanation:

The generating function of a sequence is the power series whose coefficients are the elements of the sequence. For the sequence

`1,1,1,2,3,4,5,6,...`

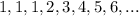
the generating function would be

`P(x)=1+x+x^2+2x^3+3x^4+4x^5+5x^6+...\\`

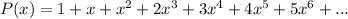
we can multiply P(x) by x to get

`xP(x)=x+x^2+x^3+2x^4+3x^5+4x^6+...`

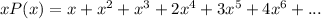
Note that

`P(x)-xP(x)=1+(2x^3-x^3)+(3x^4-2x^4)+(4x^5-3x^5)+(5x^6-4x^6)+...\\   \\=1+x^3+x^4+x^5+x^6+...=1+x^3(1+x+x^2+x^3+x^4+...)`

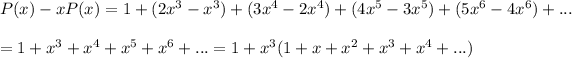
which for
`\mid x \mid < 1` can be rewritten as

`(1-x)P(x)=1+(x^3)/((1-x)) \quad \Rightarrow \\\\P(x)=(1)/((1-x))+(x^3)/((1-x)^2)`