Question

How do measurements of time differ for events in a frame of reference that moves at 50% of the speed of light relative to us? At 99.5% of the speed of light relative to us?

Answer 1

Answer with explanation:

Relation between Speed , Distance and time

Distance =Speed × Time

→It means Speed is inversely Proportional to time.

As distance will remain constant , in that frame of reference

If speed of light in a Medium

`=s=3 * 10^8 \frac{\text{meter}}{\text{second}}`

Then, time taken to cross the medium = t seconds or hours or another unit of time.

Now, If speed of light is 50% of the speed of light relative to us

That is Speed of light in another medium

`=w=(50)/(100) * 3 * 10^8\\\\=1.5 * 10^8 \frac{\text{meter}}{\text{second}}`

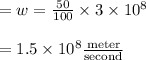
Then, time taken to cross the medium = 2t seconds or hours or another unit of time.

Using Unitary Method

`\rightarrow v_(1)* t_(1)=v_(2) * t_(2)\\\\1.\rightarrow 3 * 10^8 * t=(50)/(100) * 3 * 10^8 * t_(1)\\\\t_(1)=2t\\\\2.\rightarrow 3 * 10^8 * t=(99.5)/(100) * 3 * 10^8 * t_(2)\\\\t_(2)=(1000t)/(995)\\\\t_(2)=(200t)/(199)`

Answer 2

1.154 times the proper time

10.013 times the proper time

Explanation:

Speed of light = c

At 50% speed of light

v = 0.5c

Time dilation

`\Delta t'=\frac{\Delta t}{\sqrt{1-(v^2)/(c^2)}}\\\Rightarrow \Delta t'=\frac{\Delta t}{\sqrt{1-(0.5^2c^2)/(c^2)}}\\\Rightarrow \Delta t'=(\Delta t)/(√(1-0.25))\\\Rightarrow \Delta t'=(\Delta t)/(0.866)\\\Rightarrow \Delta t'=1.154\Delta t`

Time would differ by 1.154 times the proper time

At 99.5% speed of light

v = 0.995c

Time dilation

`\Delta t'=\frac{\Delta t}{\sqrt{1-(v^2)/(c^2)}}\\\Rightarrow \Delta t'=\frac{\Delta t}{\sqrt{1-(0.995^2c^2)/(c^2)}}\\\Rightarrow \Delta t'=(\Delta t)/(√(1-0.990025))\\\Rightarrow \Delta t'=(\Delta t)/(0.09987)}\\\Rightarrow \Delta t'=10.013\Delta t`

Time would differ by 10.013 times the proper time.