Question

The endpoints of line RS are R(1, -3) and S(4,2). Find RS

The endpoints of line CD are C(-8,-1) and D(2,4). Find CD

The midpoint of line AC is M(5,6). One endpoint is A(-3,7). Find the coordinates of endpoint C.

Answer 1

Part 1)
`RS=√(34)\ units`

Part 2)
`CD=√(125)\ units`

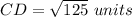
Part 3) The coordinates of endpoint C are (13,5)

Explanation:

Part 1) The endpoints of line RS are R(1, -3) and S(4,2). Find RS

we now that

the formula to calculate the distance between two points is equal to

`d=\sqrt{(y2-y1)^(2)+(x2-x1)^(2)}`

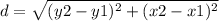
substitute the values

`RS=\sqrt{(2+3)^(2)+(4-1)^(2)}`

`RS=\sqrt{(5)^(2)+(3)^(2)}`

`RS=√(34)\ units`

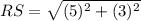
Part 2) The endpoints of line CD are C(-8,-1) and D(2,4). Find CD

we now that

the formula to calculate the distance between two points is equal to

`d=\sqrt{(y2-y1)^(2)+(x2-x1)^(2)}`

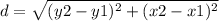
substitute the values

`CD=\sqrt{(4+1)^(2)+(2+8)^(2)}`

`CD=\sqrt{(5)^(2)+(10)^(2)}`

`CD=√(125)\ units`

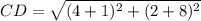
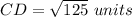
Part 3) The midpoint of line AC is M(5,6). One endpoint is A(-3,7). Find the coordinates of endpoint C

The formula to calculate the midpoint between two points is equal to

`M=((x1+x2)/(2),(y1+y2)/(2))`

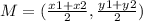
Let

(x2,y2)-------> the coordinates of point C

(x1,y1) -------> the coordinates of point A

substitute the given values

`(5,6)=((-3+x2)/(2),(7+y2)/(2))`

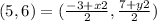
write the equations

`(-3+x2)/(2)=5\\ \\-3+x2=10\\ \\ x2=10+3\\ \\x2=13`

`(7+y2)/(2)=6\\\\ 7+y2=12\\ \\ y2=12-7\\ \\y2=5`

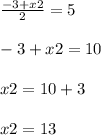
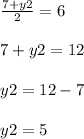
therefore

The coordinates of endpoint C are (13,5)