For this case we have to by definition, if two lines are parallel then their slopes are equal.
We find the slope of the line AB:
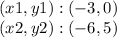
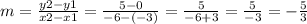
Thus, the parallel line is of the form:
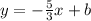
If the line passes through the origin, then we have the point (0,0):
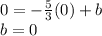
Then, the equation is:
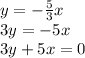
Answer:
OPTION C