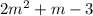Answer:
1.)
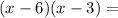
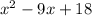
2.)

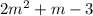
Explanation:
When solving these types of expressions we can use two different methods (both are ways to solve via distributive method), the FOIL method or the box method. I'm going to be using the FOIL method, as the box method is easier to understand visually.
FOIL stands for "First Outside Inside Last"
You multiply the first terms, then the outside terms, then the inside terms, and finally the last terms and then combine like terms.
In the first expression, (x-6)(x-3), we can use FOIL as follows:
- The First terms are: the x in (x-6) and the x in (x-3). They are the first terms in both parenthesis.
- The Outside terms are: the x in (x-6) and the -3 in (x-3). They are the outer most terms in the expression.
- The Inside terms are: the -6 in (x-6) and the x in (x-3). They are the inner most terms in the expression.
- The Last terms are: the -6 in (x-6) and the -3 in (x-3). They are the last terms in both parenthesis.
Multiply them in order, starting with
F:

O:
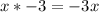
I:
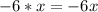
L:
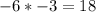
Combine like terms, in this case the like terms are: -3x and -6x, when combined, it equals -9x. Now, we put the expression in the proper order.
Your answer should be:
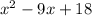
Do the same process for the next expression.
- First: 2m and m
- Outside: 2m and -1
- Inside: 3 and m
- Last: 3 and -1
Multiply:
F:
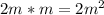
O:
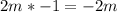
I:

L:
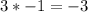
Combine like terms: -2m and 3m
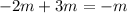
Your answer should be: