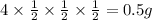Question

A sample of 4 g of cobalt isotope is produced. If the half-life of is 30 years, what will be the mass of the cobalt remaining after 90 years?

Answer 1

A sample of 4 g of cobalt isotope is produced. If the half-life of is 30 years, what will be the mass of the cobalt remaining after 90 years is 0.5 g.

Step-by-step explanation:

After 30 years the amount of cobalt isotope will be half of the original value, which is 2 g. After 60 years means, this will be decremented half of that 2 g which is 1 g. After 90 years, it will be half of the previous value that will be equal to 0.5 g.

(i.e)
`4 * (1)/(2) * (1)/(2) * (1)/(2) = 0.5 g`

Answer 2

1/2 gram.

Step-by-step explanation:

There are
`90/30 = 3` half-lives in that 90 years. The mass of this sample will have became
`(1/2)^(3) = 1/8` that of the original value by then. That is:

`\displaystyle m(90\;\text{years}) = \left((1)/(2)\right)^(90/30)\cdot m_0 = (1)/(8)* 4 = \rm (1)/(2)\; g`.