Answer: (C) 0.1591
Explanation:
Given : A manufacturer of radial tires for automobiles has extensive data to support the fact that the lifetime of their tires follows a normal distribution with
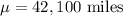
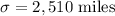
Let x be the random variable that represents the lifetime of the tires .
z-score :

For x= 44,500 miles
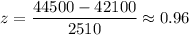
For x= 48,000 miles
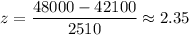
Using the standard normal distribution table , we have
The p-value :
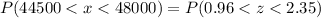

Hence, the probability that a randomly selected tire will have a lifetime of between 44,500 miles and 48,000 miles = 0.1591