Question

An ideal monatomic gas expands from an initial pressure and volume of 32 atm and 1.0 L to a final volume of 4.0 L. The initial temperature of the gas is 300 K. What are the final pressure and temperature of the gas and how much work is done by the gas if the expansion is (a) isothermal, and (b) isobaric

Answer 1

(a) P2 = 8 atm, T2 = 300 K, W = 3458.32 J

(b) P2 = 32 atm, T2 = 1200 K, W = 9696 J

Step-by-step explanation:

P1 = 32 atm

V1 = 1 L

V2 = 4 L

T1 = 300 K

(a) When the process is isothermal

The temperature remains constant, so the final temperature, T2 = 300 k

Use

P1 x V1 = P2 x V2

32 x 1 = P2 x 4

P2 = 8 atm

So, the final pressure is 8 atm and the final temperature is 300 K

Work done in isothermal expansion is given by

`W = 2.303 RT log\left ( (V_(2))/(V_(1)) \right )`

`W = 2.303 * 8.314* 300*  log 4`

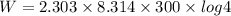
W = 3458.32 J

(b) When the process is isobaric

the pressure remains constant, so the final pressure, P2 = 32 atm

Use

V1 / T1 = V2/ T2

1 / 300 = 4 / T2

T2 = 1200 K

Work done in isobaric process

W = P (V2 - V1) = 32 x (4 - 1) = 96 atm L

W = 96 x 1.01 x 10^5 x 10^-3 = 9696 J