Question

Solve for x in the interval [0,2π]:

log₂cot(x) - 2log₄csc(2x) = log₂cos(x)

Answer 1

`x=(\pi)/(3)`

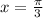
Explanation:

The given logarithmic equation is
`\log_2\cot x-2\log_4\csc 2x=\log_2\cos x`.

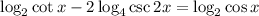We regroup to get:

`\log_2\cot x-\log_2\cos x=2\log_4\csc 2x`.

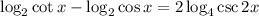Apply the quotient property of logarithms on the LHS.

`\log_2{(\cot x)/(\cos x)=2\log_4\csc 2x`.

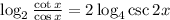Apply the power rule and change of base on the RHS

`\log_2{(1)/(\sin x)=(\log_2\csc^2 2x)/(\log_24)`.

`\log_2 \csc x=(\log_2\csc^2 2x)/(2)`.

`\log_2 \csc x=(\log_2\csc^2 2x)/(2)`.

`\log_2 \csc x=\log_2\csc 2x`.

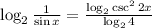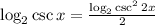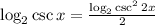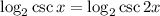We equate the arguments to get:

`\csc 2x=\csc x`

`\csc 2x-\csc x=0`

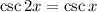
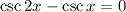
Or

`(1)/(\sin 2x)-(1)/(\sin x)=0`

`\implies (1)/(2\sin x\cos x)-(1)/(\sin x)=0`

`(1-2\cos x)/(2\sin x \cos x)=0`

`\implies 1-2\cos x=0`

`\implies \cos x=0.5`

`\implies x=(\pi)/(3),(5\pi)/(3)`

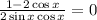
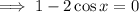
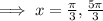
But
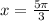`x=(5\pi)/(3)` is an extraneous solution.

Therefore
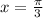`x=(\pi)/(3)` is the only solution in the interval [0,2π]