Question

An oscillating block-spring system has a mechanical energy of 3.27 J, an amplitude of 10.3 cm, and a maximum speed of 1.10 m/s. Find (a) the spring constant, (b) the mass of the block and (c) the frequency of oscillation.

Answer 1

Step-by-step explanation:

It is given that,

Mechanical energy of the block- spring system, E = 3.27 J

Amplitude of the system, A = 10.3 cm = 0.103 m

Maximum speed, v = 1.1 m/s

(a) Mechanical energy of the block spring system is given by :

`E=(1)/(2)kA^2`

`k=(2E)/(A^2)`

`k=(2* 3.27)/((0.103)^2)`

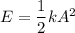
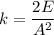
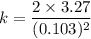
k = 616.45 N/m

(b) Velocity is maximum at the equilibrium position. The mechanical energy at the equilibrium position is given by :

`E=(1)/(2)mv^2`

`m=(2E)/(v^2)`

`m=(2* 3.27)/((1.1)^2)`

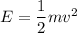
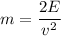
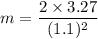
m = 5.4 kg

(c) The frequency of oscillation is :

`f=2\pi\sqrt{(m)/(k)}`

`f=2\pi* \sqrt{(5.4)/(616.45)}`

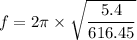
f = 0.58 Hz

Hence, this is the required solution.