Question

A​ landscaper, who just completed a rectangular flower garden measuring  15 feet in length by 6 feet in​ width, orders 1 cubic yard of premixed​ cement, all of which is to be used to create a border of uniform width around the garden. If the border is to have a depth of 2 ​inches, how wide will the border​ be? left parenthesis 1 cubic yard equals 27 cubic feet right parenthesis

Answer 1

The uniform width around the garden is 3 ft

Explanation:

Remember that

1 yd=3 ft

1 ft=12 in

1 yd³=27 ft³

step 1

Convert cubic yards to cubic feet

The volume of premixed​ cement is 27 ft³

step 2

Find the uniform width around the garden

Let

x ------> the uniform width around the garden

we know that

The area of the border is equal to

`A=[(15+2x)(6+2x)-((15)(6)]\\A=90+30x+12x+4x^(2)-90\\A=4x^(2)+42x`

To Find the volume , multiply the area by the depth

The depth is 2 inches

Convert to ft

2 in=2/12=1/6 ft

The volume is equal to

`V=[4x^(2)+42x](1/6)\\ \\V=(2)/(3)x^(2)+7x`

Remember that

`V=27\ ft^(3)`

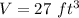
Equate the volumes

`(2)/(3)x^(2)+7x=27`

`(2)/(3)x^(2)+7x-27=0`

using a graphing tool solve the polynomial

The solution is x=3 ft

see the attached figure