Question

The law of cosines is used to find the measure of Z.

To the nearest whole degree, what is the measure of Z?

41º
47º
51º
57º

Answer 1

C. 51º

Explanation:

Answer 2

C.
`Z=51^(\circ)`

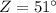
Explanation:

We have been given a triangle. We are asked to find the measure of angle Z using Law of cosines.

Law of cosines:
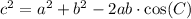`c^2=a^2+b^2-2ab\cdot \text{cos}(C)`, where, a, b and c are sides opposite to angles A, B and C respectively.

Upon substituting our given values in law of cosines, we will get:

`16^2=19^2+18^2-2(19)(18)\cdot \text{cos}(Z)`

`256=361+324-684\cdot \text{cos}(Z)`

`256=685-684\cdot \text{cos}(Z)`

`256-685=685-685-684\cdot \text{cos}(Z)`

`-429=-684\cdot \text{cos}(Z)`

`(-429)/(-684)=\frac{-684\cdot \text{cos}(Z)}{-684}`

`0.627192982456=\text{cos}(Z)`

`\text{cos}(Z)=0.627192982456`

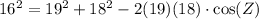
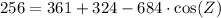
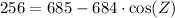
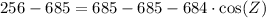
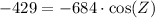
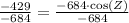
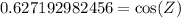
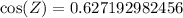
Now, we will use inverse cosine or arc-cos to solve for angle Z as:

`Z=\text{cos}^(-1)(0.627192982456)`

`Z=51.1566718^(\circ)`

`Z\approx 51^(\circ)`

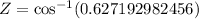
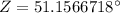
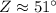
Therefore, the measure of angle Z is approximately 51 degrees.