Question

From the value Kf=1.2×109 for Ni(NH3)62+, calculate the concentration of NH3 required to just dissolve 0.016 mol of NiC2O4 (Ksp = 4×10−10) in 1.00 L of solution.

Answer 1

Answer: The concentration of
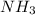`NH_3` required will be 0.285 M.

Step-by-step explanation:

To calculate the molarity of
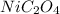`NiC_2O_4`, we use the equation:

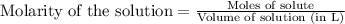
`\text{Molarity of the solution}=\frac{\text{Moles of solute}}{\text{Volume of solution (in L)}}`

Moles of
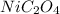`NiC_2O_4` = 0.016 moles

Volume of solution = 1 L

Putting values in above equation, we get:

`\text{Molarity of }NiC_2O_4=(0.016mol)/(1L)=0.016M`

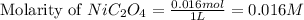
For the given chemical equations:

`NiC_2O_4(s)\rightleftharpoons Ni^(2+)(aq.)+C_2O_4^(2-)(aq.);K_(sp)=4.0* 10^(-10)`

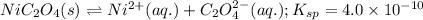
`Ni^(2+)(aq.)+6NH_3(aq.)\rightleftharpoons [Ni(NH_3)_6]^(2+)+C_2O_4^(2-)(aq.);K_f=1.2* 10^9`

Net equation:
`NiC_2O_4(s)+6NH_3(aq.)\rightleftharpoons [Ni(NH_3)_6]^(2+)+C_2O_4^(2-)(aq.);K=?`

To calculate the equilibrium constant, K for above equation, we get:

`K=K_(sp)* K_f\\K=(4.0* 10^(-10))* (1.2* 10^9)=0.48`

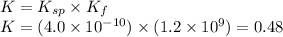
The expression for equilibrium constant of above equation is:

`K=([C_2O_4^(2-)][[Ni(NH_3)_6]^(2+)])/([NiC_2O_4][NH_3]^6)`

As,
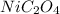`NiC_2O_4` is a solid, so its activity is taken as 1 and so for
`C_2O_4^(2-)`

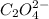
We are given:

`[[Ni(NH_3)_6]^(2+)]=0.016M`

Putting values in above equations, we get:

`0.48=(0.016)/([NH_3]^6)}`

`[NH_3]=0.285M`

Hence, the concentration of
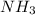`NH_3` required will be 0.285 M.