Answer: We have enough evidence to support the consumer's claim that the actual mean amount is less.
Explanation:
Let
 represents the population mean for the dispensed liquid into each paper cup.
represents the population mean for the dispensed liquid into each paper cup.
According to the claim , we have the following set of hypothesis :-
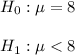
Since the alternative hypothesis is left-tailed , so the hypothesis test is left tailed test.
For sample , we have
Sample size : n=49 , which is large (n>30) , so we use z-test.
Sample mean :
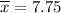
Standard deviation :
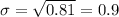
The test statistic for population mean is given by :-
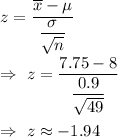
The p-value =
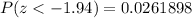
Since , the p-value is less than the significance level (0.05), so we reject the null hypothesis .
Thus , we conclude that we have enough evidence to support the alternative hypothesis i.e. the actual mean amount is less.