Answer:
The perimeter is equal to
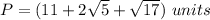 or
or
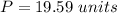
Explanation:
we have
The coordinates of the vertices are
R(-1,3), S(3,3), T(5,-1), and U(-2,-1)
plot the figure to better understand the problem
see the attached figure
we know that
The perimeter of a quadrilateral is the sum of its four length sides
so
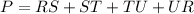
the formula to calculate the distance between two points is equal to
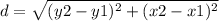
step 1
Find the distance RS
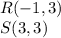
substitute the values
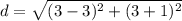

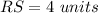
step 2
Find the distance ST
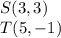
substitute the values
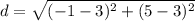
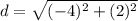
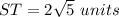
step 3
Find the distance TU
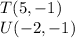
substitute the values

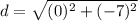
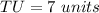
step 4
Find the distance UR
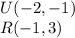
substitute the values
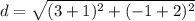
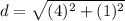
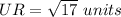
step 5
Find the perimeter
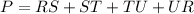
substitute the values
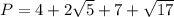
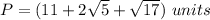 -----> exact value
-----> exact value
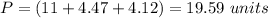 -----> approximate value
-----> approximate value