Question

The wavelength of a monochromatic source is measured with a Michelson interferometer. An interference pattern is viewed on the screen. When the movable mirror moves d = 0.14 mm, 460 fringes move past a fixed point on the screen. What is the wavelength of the light in nm?

Answer 1

Wavelength of light is 608 nm.

Step-by-step explanation:

It is given that,

Distance associated with the fringes, d = 0.14 mm = 0.00014 m

Number of fringes, m = 460

We need to find the wavelength of the light. In Michelson interferometer, the distance associated with the fringes is given by :

`d=(m\lambda)/(2)`

`\lambda=(2d)/(m)`

`\lambda=(2* 0.00014 )/(460)`

`\lambda=6.08* 10^(-7)\ m`

`\lambda=608\ nm`

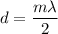
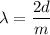
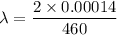
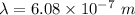
So, the wavelength of the light is 608 nm. Hence, this is the required solution.