Question

Determine if the following series converges or diverges. If it converges determine its sum.∞∑n=0 (−1)n

Answer 1

Answer: The given series neither converges nor diverges.

Step-by-step explanation: We are given to determine whether the following series converges or diverges :

`S=\sum_(n=0)^(\infty)(-1)^n.`

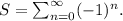
If the series converges, we are to find its sum.

The given series can be written as :

`1,~-1,~1,~-1,~1,~1,~~.~~.~~.`

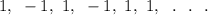
We note that the given series is a geometric one with first term 1 and common ratio given by

`r=(-1)/(1)=(1)/(-1)=~~.~~.~~.~~=-1.`

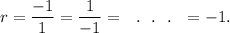
We know that a geometric series with common ratio r converges if |r| <1 and diverges if |r| > 1.

Since |r| = 1 for the given series, so the series will neither converge nor diverge.

Thus, the given series neither converges nor diverges.