Question

Calcule o valor de x

a) ㏒^ 243 = 5

b) 2 ^ 4x-4 = 512

c) 5 ^ x = 1/625

d) 2 ^ 3x-9 = 64

Answer 1

a)3

b)13/4

c)-4

d)5

NOTE: You might want to read the first section below the "Step-by-step explanation:" to see if I have interpreted your problems correctly.

Explanation:

a)

`\log_x(243)=5`

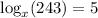
b)

`2^(4x-4)=512`

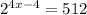
c)

`5^x=(1)/(625)`

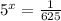
d)

`2^(3x-9)=64`

------------------------------------

a)

Let's writ ether the logarithmic form in equivalent exponential form:

`x^5=243`

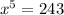
To solve this we need to take the fifth root of both sides:

`x=243^(1)/(5)`

`x=3`

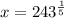
b)

We are going to write both sides so their bases are 2.

The left hand side is already base 2 so we are not doing anything to that side.

The 512 however can be written as 2^9.

So we have:

`2^(4x-4)=2^(9)`

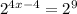
Since the bases are the same, the only thing we can do is set the exponents equal so those are the same as well.

`4x-4=9`

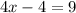
Add 4 on both sides:

`4x=13`

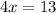
Divide both sides by 4:

`x=(13)/(4)`

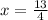
c)

We are going to write both sides so their bases are 5.

This does not effect left hand side since the base is already 5 on that side.

I know 5^4=625 so 5^(-4)=1/625.

So we have:

`5^x=5^(-4)`

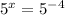
This implies
`x=-4`.

d)

We are going to write both sides so they have base 2.

Left hand side is done. Let's move on to the right. 64=2^6.

`2^(3x-9)=2^6`.

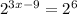This implies:

`3x-9=6`

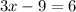
Add 9 on both sides:

`3x=15`

Divide both sides by 3:

`x=(15)/(3)`

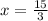
Simplify:

`x=5`