Question

The probability that a person with certain symptoms has hepatitis is 0.75. The blood test used to confirm this diagnosis gives positive results for 86?% of people with the disease and 5?% of those without the disease. What is the probability that an individual who has the symptoms and who reacts positively to the test actually has? hepatitis?

Answer 1

0.981

Explanation:

We have to find the probability that an individual who has the symptoms and who reacts positively to the test has hepatitis

Let
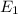`E_1`be the event that denotes the symptoms has hepatitis and
`E_2` denotes the symptoms have not hepatitis

Let A be the event the denotes the blood test result positive

We have to find the value of
`P(E_1/A)`

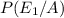
We have
`P(E_1)=0.75`

`P(E_2)=1-0.75=0.25`

`P(A/E_1)=86%=0.86`

`P(A/E_2)=5%=0.05`

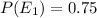
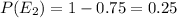
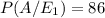
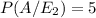
Using formula

`P(E_1/A)=(P(E_1)* P(A/E_1))/(P(E_1)\cdotP(A/E_1)+P(E_2)\cdotP(A/E_2)`

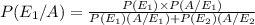
Substitute the values in the given formula

Then,we get

`P(E_1/A)=(0.75* .86)/(0.75*0.86+0.25* 0.05)`

`P(E_1/A)=(0.645)/(0.6575)=0.9809`

`P(E_1/A)=0.981`

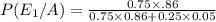
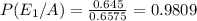
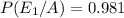
Hence, the probability that an individual who has the symptoms and who reacts positively to the test actually has hepatitis =0.981