Answer:

Explanation:
We know the function

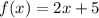
We want to find
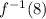
Remember that if we have a function
 and its inverse
and its inverse
 , then the domain of the function
, then the domain of the function
 will be the range of the function
will be the range of the function
 .
.
This means that to find
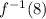 we must look for:
we must look for:
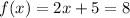
We solve the equation for x.
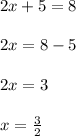
So:
