Question

In △ABC, m∠A=72

△
A
B
C
,

m
∠
A
=
72
°, c=61
c
=
61
, and m∠B=16
m
∠
B
=
16
°. Find the perimeter of the triangle.

Answer 1

135.86≈ 136

Explanation:

As Given in figure 1:

In ΔABC, m∠A = 72°, m∠B = 16° and c = 61 , where a, b, and c are lengths of side of ΔABC.

To find: Perimeter of triangle = ?

Sol: In ΔABC,

m∠A + m∠B + m∠C = 180° (sum of angles of a triangle)

m∠C = 180° - (72° + 16°)

m∠C = 92°

Now Using Sine Rule:

`(a)/(SinA) = (b)/(SInB) = (c)/(SInC)`

`(a)/(Sin 72^(\circ)) = (b)/(Sin 16^(\circ)) = (61)/(SIn92^(\circ))`

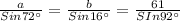
Now,
`(a)/(sin72^(\circ)) = (61)/(Sin92^(\circ))`

∴
`a = (61 * Sin 72^(\circ))/(Sin92^(\circ)) = (61 * 0.951)/(0.999) = (58.011)/(0.999) = 58.07`

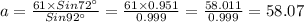
In the same way,
`(b)/(sin 16^(\circ)) = (61)/(Sin92^(\circ))`

∴
`b = (61 * Sin 16^(\circ))/(Sin92^(\circ)) = (61 * 0.275)/(0.999) = (16.775)/(0.999) = 16.79`

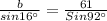
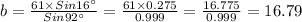
Therefore, a = 58.07 ≈ 58, b = 16.79 ≈ 17 and c = 61

Now, Perimeter of ΔABC = a + b + c = 58 + 17 + 61 = 136