That is a question about triangles.
In my answer I will show 2 ways to solve that question, ok? Let's go.
First way - Pythagoras's theorem
The line segment x divide the biggest triangle in 2 equals right triangles.
Let's choose one of them and note that is a triangule with hypotenuse equals to 8 and one cathetus equals to half of 8.
The Pythagoras's theorem says that:
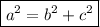
a is the hypotenuse and b and c are cathetus.
So, in our case, we know the hypotenuse and one cathetus, let's substitute that in the expression:
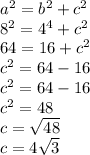
Therefore, the value of x is
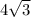 .
.
Second way - The equilateral triangle height
That way to solve the question is a consequence of the previous way.
That triangle has all the sides equals, so it is a equilateral triangle.The line segment x is the height of that triangle. And we can find the equilateral triangle height using that expression:
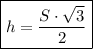
h is the height and S is the triangle's side.
So, we know that the side of our triangle is 8. Let's change S value in the expression:

Thus, the value of x is
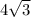 .
.
Note that in the 2 ways we find the same result, so that answer is correct.
I hope I've helped. :D
Enjoy your studies! \o/