Answer:
7
Explanation:
The average rate of change here, since this is not a line, is the slope of the secant line that is connecting those 2 points. We can find that average rate of change by finding the y coordinates that go with each of those x coordinates and then applying the slope formula. For g(0):
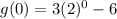
Anything raised to the power of 0 is 1, so we have then:
g(0) = 3(1) - 6 so
g(0) = -3 and the coordinate pair is (0, -3)
For g(3):
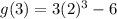 so
so
g(3) = 18 and the coordinate pair is (3, 18).
Now we plug those into the slope formula:

So the averagee rate of change, which is also the slope, between those 2 points is 7