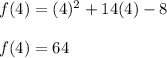Answer: The remainder is 64.
Explanation:
By definition, when we divide a polynomial f(x) by
 the remainder will be:
the remainder will be:
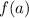
In this case we know that f(x) is:
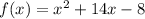
And we need to find the remainder when it is divided by
 .
.
Therefore, substituting
 into f(x), we get that the remainder is:
into f(x), we get that the remainder is: