Question

In a large population, 70 % of the people have been vaccinated. If 4 people are randomly selected, what is the probability that AT LEAST ONE of them has been vaccinated?

Answer 1

Answer: 0.9919

Explanation:

Binomial probability formula :-

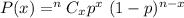`P(x)=^nC_xp^x\ (1-p)^(n-x)`, where n is total number of trials , P(x) is the probability of getting success in x trials and p is the probability of getting success in each trial.

Given : The proportion of the people have been vaccinated: p=0.7

If 4 people are randomly selected, then the probability that at least one of them has been vaccinated will be :-

`P(X\geq1)=1-P(0)`

`=1-^4^C_0(0.7)^0(0.3)^4\\\\=1-(0.3)^4=0.9919`

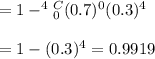
Hence, the probability that at least one of them has been vaccinated =0.9919

Answer 2

The probability that at least one of the 4 people has been vaccinated is 0.9919.

Explanation:

It is given that in a large population, 70 % of the people have been vaccinated. So, the probability of success is

`p=(70)/(100)=0.7`

Probability of failure is

`q=1-p=1-0.7`

According to binomial distribution.

`P(x=r)=^nC_rp^rq^(n-r)`

We need to find the probability that at least one of the 4 people has been vaccinated.

`P(x\geq 1)=1-P(x<1)`

`P(x\geq 1)=1-P(x=1)`

`P=1-^4C_0(0.7)^0(0.3)^(4-0)`

`P=1-1(0.0081)`

`P=0.9919`

Therefore the probability that at least one of the 4 people has been vaccinated is 0.9919.